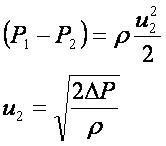ReStackor uses fundamental fluid dynamics to accurately compute flow through the clicker bleed circuits
Bernoulli Equation
The Bernoulli equation computes the pressure required to force fluid flow through a flow restriction. That pressure is computed in terms of force and flow acceleration using Newtons fundamental relationship:
For a fluid flow the force is the pressure times the area (F=PA), the mass is the fluid contained within a small axial slice of the flow (m= rho A dx) and the acceleration is the change in fluid velocity (a=du/dt). With these definitions Newtons equation becomes:
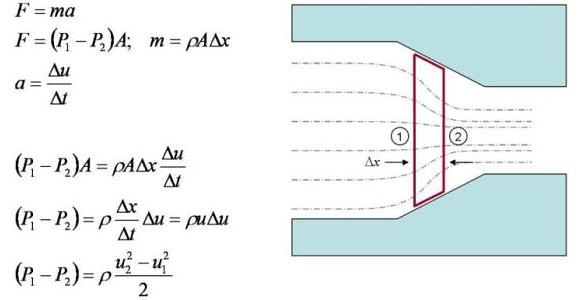
![]()
Bernoulli Equation Developed in 1738
For flow from a reservoir into an orifice the initial velocity is zero (u[1]= 0) and the above equation becomes:
This is the well know Bernoulli equation developed in 1738. The equation relates flow velocity to the pressure and force required to establish that velocity. For tuning of a suspension system this equation is more intuitive written the other way:
The pressure force generated by a shock absorber is proportional to the velocity squared. If you hit a bump that causes the suspension to move twice as fast the flow resistance and pressure drop through an orifice increases by a factor of four. This rapid increase in pressure for small changes in velocity is why shock absorbers use shim stacks to control the valve flow area. At high velocities deflection of the shims increases the valve flow area. The larger area reduces the fluid velocity, acceleration and pressure drop required to push the flow through the valve gap. The variable area of the shim stack keeps the pressure from spiking within the shock absorber.
Through control of the shim stack stiffness you control the damping forces produced in the shock absorber.
![]()
Viscosity effects
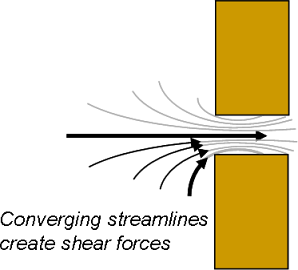
High viscosity fluids increase the pressure drop through an orifice. Flow approaching an orifice along the centerline flows directly in, flow approaching from the side has to turn into the orifice throat. Turning of the flow entering from the side bends the streamlines and creates velocity gradients across the entrance flow. The stream line velocity differences generate shear forces as the high velocity centerline flow tries to drag the lower velocity perimeter flow along with it. While these forces are small it is these shear forces that create the differences in the flow resistance when you change from 5wt to higher viscosity 10wt oil.
High oil viscosities increase shear losses in orifice entrance flows.
Turning of the streamlines at the orifice entrance also creates a fluid dynamic flow restriction. The low velocity side entrance flow is still turning as it enters the orifice. This gives the perimeter flow a lower axial velocity and an “effective” orifice throat flow area that is smaller then the geometric area of the orifice. The amount of restriction is dependant on the flow velocity, fluid momentum, entrance geometry and viscosity of the fluid. ReStackor calculations account for these effects using Reynolds number relationships that describe the effective flow area and flow resistance as a function of fluid viscosity, flow velocity and the geometry of the orifice entrance.
![]()
So does any of this really work?
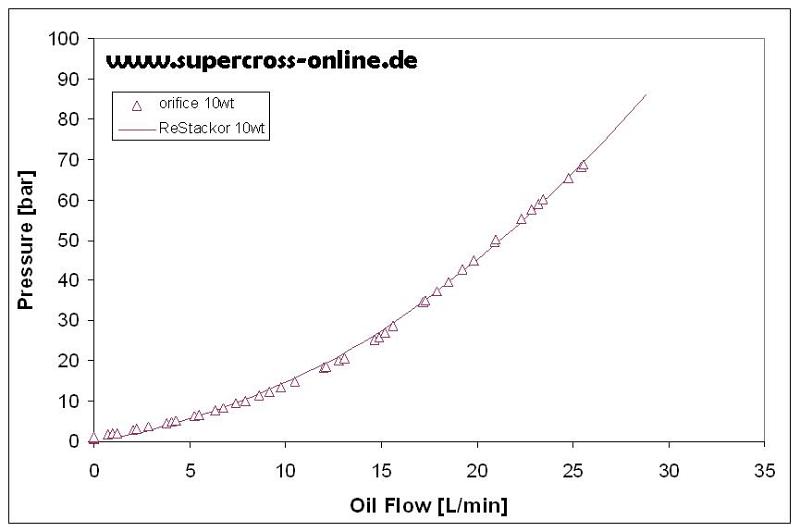
The fluid dynamic relationships used in ReStackor have been tested against real world data for real pressure drops measured in real suspension hardware at www.supercross-online.de. Example supercross-online data for bleed circuit pressure drops is shown below in comparison to ReStackor pro calculations. ReStackor matches the supercross-online orifice flow data. The 30 L/min flow rate of this bleed circuit test data is equivalent to wheel speeds of 600 in/sec in an actual suspension system. The test data spans the range of low speed to high speed suspension operation and demonstrates that ReStackor calculations are capable of accurately modeling flows through the bleed circuit over the entire range of suspension speeds. This data comparison has very important implications for suspension tuning as ReStackor uses clicker settings to relate changes in shim stack stiffness and help you setup your suspension in terms of clicker settings and the forces you can actually feel when you ride your bike.
ReStackor orifice flow calculations accurately match dyno test data.
![]()
Viscosity Effects on Bleed Circuit Flow
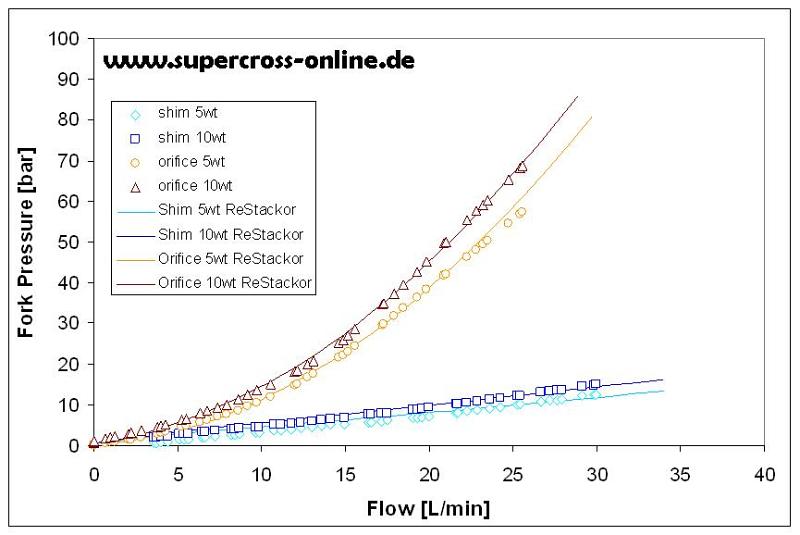
Testing at supercross-online has also evaluated effects of changes in oil viscosity on flow rates through the orifice bleed circuits. ReStackor pro is capable of accurately computing effects of fluid viscosity changes on the flow rate through orifice bleed circuits and the change in damping force from the clicker bleed circuit as well as the effect of oil viscosity changes on flows through the valve stack. This gives you the capability to tune the suspension in terms of clicker settings or the changes in damping force caused by oil viscosity changes.
ReStackor calculations accurately match dyno test data measuring oil viscosity effects on bleed circuit flow rates.